Buildings

Arun Ram
Department of Mathematics and Statistics
University of Melbourne
Parkville, VIC 3010 Australia
aram@unimelb.edu.au

Last updates: 11 August 2012

The concept

A building is an axiomatization of the flag variety $G/B$ of a Kac-Moody group $G$. The advantages of the building point of view are

1. a "conceptual picture" of the geometry of the flag variety
2. a good view of the "independence" of the geometry from the underlying field,
3. a powerful way to work with tori by viewing them as apartments.

The disadvantage is that the axioms do not allow for certain spaces that ought to be considered as flag varieties of "Lie type" groups and this dichotomy between buildings and Lie type groups is unhealthy.

Let $G$ be a group, $B$ a subgroup of $G$ and let $W$ be an index set for the double cosets of $B$ in $G$ so that $$G=\underset{w\in W}{⨆}BwB.$$

Morally, the building of $(G,B)$ is the set $ℬ=G/B$ with the function $\delta :ℬ\times ℬ\to W$ given by $$\delta (g_{1}B,g_{2}B)=w,\text{if}B{g}_1{g}_2^{-1}B=BwB.$$ In the case when $B$ is a Borel subgroup of a reductive algebraic group then $W$ is a group with a distinguished set of generators: a Coxeter group. The usual approach to buildings is to axiomatize $ℬ$ building in terms of the group $W$ and its special choice of generators.

The definition of a building

A chamber system $ℬ$, or flag system on a set $S=\{s_1,\dots ,s_n\}$ is $$\text{a set}ℬ\text{with given equivalence relations}{\sim }_j\text{on}ℬ$$ indexed by the elements $s_1,\dots ,s_n$ of $S$. The set $ℬ$ is the set of chambers or flags and the relations ${\sim }_j$ are the adjacency relations. For a fixed chamber $c\in ℬ$, the chambers $j$-adjacent to $c$ look lik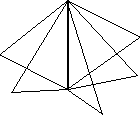e When $\mathrm{Card}\{d\mid d\ne c,d{\sim }_{i}c\}$ is the same for all $i$ and $c$, $$q=\mathrm{Card}\{d\mid d\ne c,d{\sim }_{i}c\}\text{is the <strong>thickness</strong> of}ℬ.$$ A gallery of type $i_1,\dots ,i_{\ell }$ is a sequence $$c_1{\sim }_{i_1}\phantom{i}{c}_2{\sim }_{i_2}\cdots {\sim }_{i_l}\phantom{i}{c}_{\ell }\text{of chambers such that}{c}_k\ne c_{k+1}.$$ A Coxeter group is a group $W$ with a given presentation by generators $s_1,\dots ,s_n$, and relations $$s_j^2=1\text{and}{\left(s_i{s}_j\right)}^{m_{ij}}=1,$$ where $m_{ij}$ is the order of $s_i{s}_j$ ($m_{ij}=\infty$ is allowed). Hence, the data of a Coxeter group is the set $$S=\{s_1,\dots ,s_n\}\text{and the orders}{m}_{ij}\text{of the products}{s}_i{s}_j.$$ A building of type $W$ is a chamber system $ℬ$ over $S$ with a function $\delta :ℬ\times ℬ\to W$ such that

1. If $s_i\in S$ and $c\in ℬ$ then there exists $c\prime \in ℬ$ with $c\prime {\sim }_i\phantom{,}c$.
2. If $s_{i_1}\cdots s_{i_{\ell }}\in W$ is a reduced expression and there is a gallery of type $i_1,\dots ,i_{\ell }$ from $c$ to $d$ then $\delta (c,d)=s_{i_1}\cdots s_{i_{\ell }}$.

The relative position of $c$ and $d$ is $\delta (c,d)$ and the adjacency relations in $ℬ$ are recovered from the fact that $$\delta (c,d)=s_j\text{if and only if}c\phantom{i}{\sim }_j\phantom{,}d.$$ If $W$ is finite and crystallographic $ℬ$ is a spherical building and if $W$ is an affine Weyl group $ℬ$ is an affine building.

A geometric realization of $ℬ$ is a realization of the simplicial complex which has

• vertices: $R_{I-\left\{i\right\}}\left(c\right)=\{\text{galleries from}c\text{with adjacency labels contained in}I-\{i\left\}\right\}$,
• simplices: $R_J\left(c\right)=\left\{\text{galleries from}c\text{with adjacency labels contained in}J\right\}\}$,

where $J$ is a subset of $I$.

Apartments and retraction

Let $W$ be a Coxeter group. The Coxeter complex of $W$ is the building $W$ given by $$w{\sim }_j\phantom{,}w{s}_j\text{and}\delta (u,v)=u^{-1}v.$$ A geometric realization of $W$ is the reflection representation ${𝔥}^{*}$ of $W$ where the chambers are the fundamental regions for the action of $W$.

Let $ℬ$ be a building of type $W$. An apartment is a sub-chamber system of $ℬ$ isomorphic to the Coxeter complex $W$. $$\text{PICTURE}$$

1. If $c_1,c_2\in ℬ$ then there exists an apartment $𝔱$ such that $c_1,c_2\in 𝔱$.
2. If $𝔱,𝔱\prime$ are apartments such that $𝔱\cap 𝔱\prime \ne \varnothing$ then there is an isomorphism $\psi :𝔱\stackrel{\sim }{⟶}𝔱\prime$ such that $\psi {\mid }_{𝔱\cap 𝔱\prime }=\mathrm{id}$.
3. Apartments are convex: If a chamber $c\prime$ lies on a minimal length gallery joining chambers $c$ and $d$ then $c\prime$ lies in every apartment containing $c$ and $d$.

Reformulating the axioms of a building in terms of apartment, a building of type $W$ is a simplicial complex $ℬ$ with a collection $𝔗$ of subcomplexes, the apartments of $ℬ$, such that

1. $ℬ\ne \varnothing$,
2. If $𝔱\in 𝔗$ then $𝔱\cong W$,
3. If $c_1,c_2\in ℬ$ then there exists $𝔱\in 𝔗$ such that $c_1\in 𝔱$ and $c_2\in 𝔱$,
4. If ${𝔱}_1,{𝔱}_2\in 𝔗$ and ${𝔱}_1\cap {𝔱}_2\ne \varnothing$ then there exists an isomorphism $\psi :{𝔱}_1\stackrel{\sim }{⟶}{𝔱}_2$ such that $\psi {\mid }_{{𝔱}_1\cap {𝔱}_2}=\mathrm{id}$.

Let $𝔱$ be an apartment and $c$ a chamber in $𝔱$. The retraction onto $𝔱$ centered at $c$ is the map $${\rho }_{𝔱,c}:ℬ⟶𝔱,\text{given by}{\rho }_{𝔱,c}\left(d\right)=\psi \left(d\right),$$ where $𝔱\prime$ is an apartment containing both $c$ and $d$ and $\psi :𝔱\prime ⟶𝔱$ is an isomorphism.

Affine buildings

Let $ℐ$ be an affine building. An alcove $w\in \stackrel{\sim }{W}$ is dominant if it is on the positive side of $H_{\alpha }$ for all $\alpha \in R^{+}$. The dominant chamber is $$C=\{wI\mid w\text{is dominant}\}\text{PICTURE}$$ A sector is a subchamber system of $ℐ$ isomorphic to $C$.

1. If $C$ is a sector and $c$ is a chamber in $ℐ$ then there exists an apartment $𝔱$ containing $c$ and a subsector of $C$.
2. If $C$ and $D$ are sectors, then there exist subsectors $C\prime \subseteq C$ and $D\prime \subseteq D$ which lie in a common apartment.

Let $𝔱$ be an apartment and $C$ a sector in $𝔱$. The retraction onto $𝔱$ centered at $C$ is the map $${\rho }_{𝔱,C}:ℐ⟶𝔱\text{given by}{\rho }_{𝔱,C}\left(d\right)=\psi \left(d\right),$$ where $𝔱\prime$ is an apartment containing $d$ and a subsector of $C$ and $\psi :𝔱\prime ⟶𝔱$ is an isomorphism.

The spherical building at infinity or boundary of $ℐ$ is the set $\partial ℐ$ of equivalence classes of sectors with respect to the equivalence relation where $D_1$ and $D_2$ are parallel $$D_1\Vert D_2\text{if}{D}_1\cap D_2$$ contains a sector.

Dictionary to algebraic groups

Let $G$ be a linear algebraic group. Let $W$ be the Weyl group and let $B$ be a Borel subgroup of $G$. The $$\text{<strong>flag variety</strong>}ℬ=\left\{\text{Borel subgroups of}G\right\}\simeq G/B$$ is a (spherical) building of type $W$ such that $$\begin{array}{ccc}\hfill \left\{\text{simplices in}ℬ\right\}& =& \left\{\text{proper parabolic subgroups in}G\right\}\hfill \\ \hfill \left\{\text{chambers in}ℬ\right\}& =& \left\{\text{minimal parabolic subgroups in}G\right\}\hfill \\ \hfill \left\{\text{vertices in}ℬ\right\}& =& \left\{\text{maximal parabolic subgroups in}G\right\}\hfill \\ \hfill \left\{\text{apartments in}ℬ\right\}& \leftrightarrow & \left\{\text{maximal split tori in}G\right\}\hfill \end{array}$$ so that $$\left\{\text{simplices in an apartment}\right\}=\left\{\text{parabolics}P\text{such that}P\supseteq T\right\}.$$ Let $G\left(𝔽\right)$ be the group $G$ over the field $𝔽$, $\stackrel{\sim }{W}$ the affine Weyl group, and let $I$ be an Iwahori subgroup of $G\left(𝔽\right)$. The $$\text{<strong>affine flag variety</strong>}ℐ=\left\{\text{Iwahori subgroups of}G\right(𝔽\left)\right\}\simeq G\left(𝔽\right)/I$$ is an (affine) building of type $\stackrel{\sim }{W}$ with $$\begin{array}{ccc}\left\{\text{simplices in}ℐ\right\}& =& \left\{\text{proper parahoric subgroups in}G\right(𝔽\left)\right\}\hfill \\ \left\{\text{sectors in}ℐ\right\}& \leftrightarrow & \left\{\text{proper parabolics in}G\right(𝔽\left)\right\}\hfill \end{array}$$ In $G/I$ our favourite chamber, vertex, apartment and sector are $$I,0=K,𝔥=\{\mathrm{wI}\mid w\in \stackrel{\sim }{W}\},U^{-}=\{\mathrm{wI}\mid w\text{is dominant}\},$$ respectively. If $U^{-}$ is the favourite sector, its equivalence class $\left[U^{-}\right]$ has stabiliser $B\left(𝔽\right)$ and $$\begin{array}{ccc}ℬ& \stackrel{\sim }{⟶}& \partial ℐ\\ gB& \mapsto & g\left[U^{-}\right]\end{array}$$ is a bijection between the building $ℬ=G\left(𝔽\right)/B\left(𝔽\right)$ and $\partial ℐ$.

Let $v,w\in \stackrel{\sim }{W}$. Then $$\begin{array}{cc}IwI=\{gI\mid \delta (I,gI)=w\},& U^{-}vI=\{hI\mid {\rho }_{𝔥,w_{0}C}(hI)=vI\},\\ IwI=\{gI\mid {\rho }_{𝔥,I}(gI)=wI\},& U_{w}vI=\{hI\mid {\rho }_{𝔥,\mathrm{wC}}(gI)=vI\}.\end{array}$$

On the classification

Most buildings are constructed as in (???) and (???). There are only a few "exotic cases" when the rank is 2 or 3. The classification of spherical buildings of rank ≥ 3 [?] and of affine buildings of rank ≥ 4 [Tits, Como] says that they are the buildings corresponding to BN-pairs in untwisted or twisted Chevalley groups over finite fields, local fields or power series fields.

• A type $A_1$ building is a set of chambers, all pairwise adjacent.
• Buildings of type $I_2\left(m\right)$ are in bijection with generalised $m$-gons (see [Batten] and [Ronan, Proposition 3.2]). There is no known classification, even for $m=3$, where they are in bijection with combinatorial projective planes. The known examples are given in [Batten].
• The buildings of type ${\stackrel{\sim }{A}}_1=I_2\left(\infty \right)$ are trees such that every vertex has valency ≥ 2.
• There is a "free construction" of rank 3 affine buildings given by Ronan [R2] where the building is built outwards from a chamber by gluing together rank 2 spherical buildings. The freedom in the choices of the spherical buildings in this construction illustrates that a classification of rank 3 affine buildings in the spirit of the rank ≥ 4 classification is impossible.

For $m\ge 2$, a generalised m-gon is a connected graph $\Gamma$ satisfying

1. the vertices of $\Gamma$ can be partitioned into "type 1" and "type 2" such that no two vertices of the same type are connected by an edge,
2. the maximum distance between two vertices of $\Gamma$ is $m$,
3. the length of the shortest circuit in $\Gamma$ is $m$.

Generalised $m$-gons are the same as buildings of type $I_2\left(m\right)$ by taking chambers to be the edges of the generalised $m$-gon, and declaring chambers $i$-adjacent ($i=\mathrm{1,2}$) if they share a type $i$ vertex (see [Ronan, Proposition 3.2]).

A combinatorial projective plane consists of a set of lines $L$, a set of points $P$, and an incidence relation $\in$ between points and lines (a subset of $L\times P$; write $p\in \ell$ if $p$ is incident to $\ell$) such that

• If $p_1,p_2\in P$ then there exists a unique $\ell \in L$ such that $p_1\in \ell$ and $p_2\in \ell$,
• If ${\ell }_1,{\ell }_2\in L$ then there exists a unique $p\in P$ such that $p\in {\ell }_1$ and $p\in {\ell }_2$,
• There exist $p_1,p_2,p_3,p_4\in P$ such that there is no $\ell \in L$ containing three of $p_1,p_2,p_3,p_4$.

Combinatorial projective planes are the same as generalised 3-gons by setting $P$ to be the vertices of type 1, $L$ to be the vertices of type 2 and letting the edges specify the incidence relation.

Notes and References

This page is the result of joint work with James Parkison in 2006. A significant part of this page overlaps with a file buildings12-18-06.tex in Work2007/Bites2007.

References

[Br] K. Brown, Buildings, Springer-Verlag, New York, 2002. ISBN: ?????? MR??????.

[Ro] M. Ronan, Lectures on Buildings, Perspectives on Mathematics, Academic Press, 1989 ISBN: ?????? MR??????.

[Ti] J. Tits, Buildings of Spherical Type and Finite BN-pairs, Lecture Notes in Mathematics, Springer-Verlag, volume 386, 1974. ISBN: ?????? MR??????.

page history